I don't know...

On another subject however,

So far we have only looked at applying geometry to a small piece of paper. That's good for making plans and so forth, but these methods are not practical or even very accurate should you wish to lay out a full sized building.

So how do we do this?

More importantly, how did they do this 500, 1000, or even 2000 years ago.

How did the Egyptians do this to create things as accurate as the pyramids?

How did the Romans do this to create their great works, and to make their system of roads?

To transfer the geometry of the paper to reality, we need a number of things.

We need some way of laying out a straight line over a very long distance, some sort of surveying device.

We need a way to make things plumb. Plumb lines and plumb bobs do the trick here.

We need a way to make things level. And that's the tricky part. Once again, the plumb line can be our friend.

For the first task, laying out long straight lines, there is an ancient device that the Romans called the groma
The groma consists of a long pole driven into the ground, on the top of which two beams crossing each other at right angles (making a + shape) are mounted offset from the beam on a swiveling arm. Under the center of the + there is a plumb line to be lined up with a datum point. Each of the four arms has a plumb line as well. The whole assembly looks like this:


to establish a straight line, one man sights down 3 of the plumb lines (the North-South lines and the center line) while another marks off stakes according to the directions of the surveyor. And so incredibility accurate lines can be made. In addition, the groma allows us to make a line at a right angle to the first line using the east-west points.

For the second need, a plumb line will tell us the perfect vertical, however we will need a way of applying this vertical to a post, for example. We cannot simply place the plumb line against the side of the post and expect an accurate reading. Instead, we mount the plumb line to a board with a straight line marked parallel to its edges, and use it to set the post to plumb.

So how about level?
The level as we have it today is the result of millenia of development. The simplest level is a long piece of wood with a chanel cut into its center that is filled with water. This is not the most accurate method, but works for approximations. It can be accurate on a large scale, level foundations can be established by filling the foundation ditch with water and marking the water line to use as a reference point.

[img]

A better method for smaller things is to use the plumb bob again. To do this, we exploit the nature of the isosceles triangle. A plumb line is attached at the point of an isosceles triangle, and when the line lines up with the center of the bottom side then the bottom side of the triangle is level. This might be placed against a level line on a timber, for example.



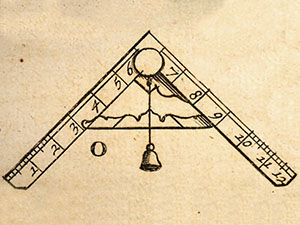
Some variations of the plumb level

what would appear to be a French variation of an ancient Roman leveling device.

So with these tools we can create straight lines to create our building plan, and then we can erect our frames true and square.

The groma can be used in conjunction with a rope, stakes, or other methods of establishing the straight line. when marking off distances with a large compass or trammel it can be used to ensure you stay in line. For the first line of a structure it is unnecessary, but from then on its use makes the whole task much easier. It eliminates the need to do extensive geometric operations to establish right angle and parallel lines.

The groma is believed to have been invented by the Egyptians, along with the plumb line, plumb level, water level, measuring rope, and other such tools. It survived in various forms for thousands of years.

The plumb bob was the ultimate tool of the ancient builder and surveyor. The Egyptians discovered ways to use the plumb line in about every operation from establishing a level surface to making straight lines and complex angles. The plumb line is a reference point that can always be trusted to be exactly true. When combined with the geometry of triangles, the plumb line can be used to establish a wide variety of angles, creating the first protractor. A graduated disk with two sight points attached to the top of a groma can be used to find angles off of the straight or perpendicular lines as well.